Modulus Argument Form
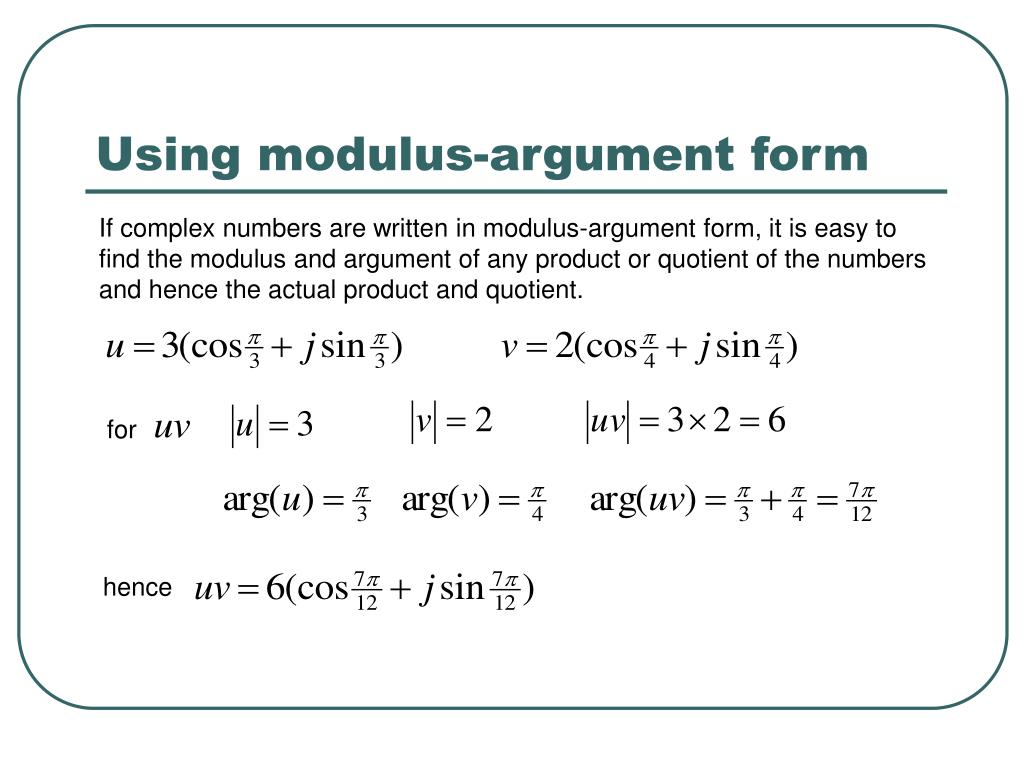
Modulus Argument Form - (a) and (b) and (c). By giving your answers , find: Among the two forms of these numbers, one form is z = a + bi, where i. I) 1 + i tan θ, ii) 1 + i cot θ, iii) 1 sin θ + 1 cos θ i. Web modulus and argument a complex number is written in the formim z=x+ iy: Web introduction complex numbers are imaginary numbers, and the complex plane represents these numbers. The complex number is said to be in cartesian form. (b) hence simplify each of the. Theargumentofzis x re y argz= = arctan:. The complex number z = 4 + 3i.
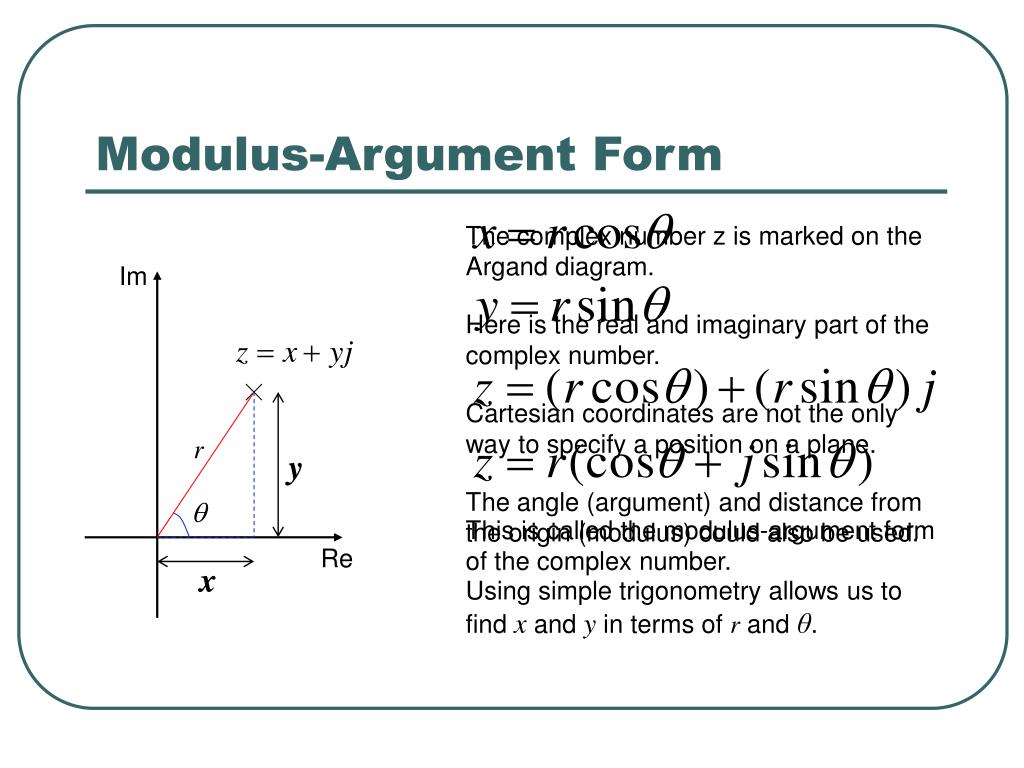
If the z = a +bi is a complex. ⇒ also see our notes on: Web modulus and argument a complex number is written in the formim z=x+ iy: The formula |z| = √ (x 2 +y 2 ) gives the modulus of a complex number z = x + iy, denoted by |z|, where x is the real component and y is the. Theargumentofzis x re y argz= = arctan:. I) 1 + i tan θ, ii) 1 + i cot θ, iii) 1 sin θ + 1 cos θ i. Among the two forms of these numbers, one form is z = a + bi, where i. Web the modulus (also known as the magnitude or absolute value) of a complex number is a scalar value that represents the distance of the complex number from the origin on the. Using the formula, we have: The complex number is said to be in cartesian form.
Among the two forms of these numbers, one form is z = a + bi, where i. By giving your answers , find: The complex number is said to be in cartesian form. (b) hence simplify each of the. Web the modulus and argument are fairly simple to calculate using trigonometry. Using the formula, we have: (a) and (b) and (c). Themodulusofzis 6 z=x+ iyy u 3 jzj =r=px2+y2: The formula |z| = √ (x 2 +y 2 ) gives the modulus of a complex number z = x + iy, denoted by |z|, where x is the real component and y is the. ⇒ also see our notes on:
Modulus, Argument and Conjugate YouTube
I) 1 + i tan θ, ii) 1 + i cot θ, iii) 1 sin θ + 1 cos θ i. Web the modulus is the length of the line segment connecting the point in the graph to the origin. We can join this point to the origin with a line segment. Examples of finding the modulus and argument |.
All About Complex Numbers in Modulus Argument Form YouTube
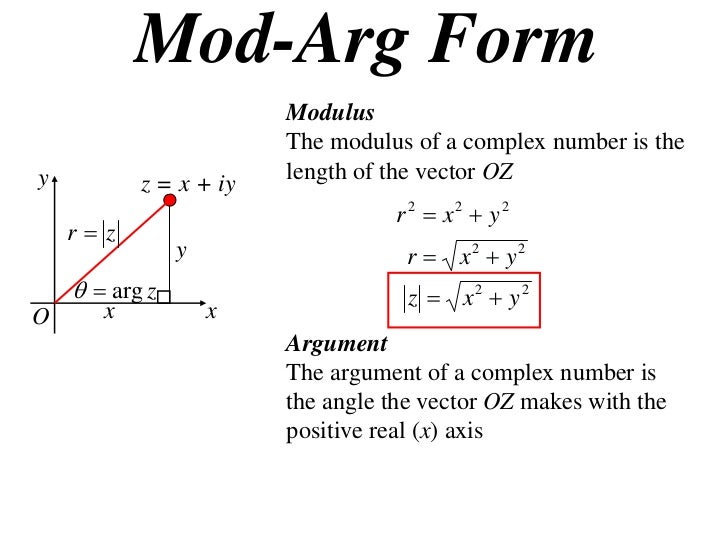
Modulus ( magnitude ) the modulus or magnitude of a complex number ( denoted by ∣z∣ ), is the distance between the origin and that number. Web introduction complex numbers are imaginary numbers, and the complex plane represents these numbers. | z | = a 2 + b 2 | 3 + 3 3 i | = 3 2 +.
PPT Complex Numbers PowerPoint Presentation, free download ID956714
Find the modulus and argument of z = 4 + 3i. We can join this point to the origin with a line segment. Web modulus and argument definition any complex number z z can be represented by a point on an argand diagram. There are, however, other ways to write a complex number, such as in modulus. Web the modulus.
X2 T01 03 argand diagram
| z | = a 2 + b 2 | 3 + 3 3 i | = 3 2 + ( 3 3) 2 | 3 + 3 3 i |. Web modulus and argument definition any complex number z z can be represented by a point on an argand diagram. Web complex number modulus formula. If the z =.
Complex Number 2 2i convert to Trigonometric Polar modulus argument
Web the modulus (also known as the magnitude or absolute value) of a complex number is a scalar value that represents the distance of the complex number from the origin on the. Web introduction complex numbers are imaginary numbers, and the complex plane represents these numbers. Web modulus and argument a complex number is written in the formim z=x+ iy:.
HSC 4U Maths Complex Numbers Changing to ModulusArgument Form YouTube
Web when an argument is outside , add or subtract multiples of until the angle falls within the required range. Web complex number modulus formula. (b) hence simplify each of the. ⇒ also see our notes on: Web the modulus (also known as the magnitude or absolute value) of a complex number is a scalar value that represents the distance.
Chapter 3 Further Complex Numbers Write down a complex number, z, in
Themodulusofzis 6 z=x+ iyy u 3 jzj =r=px2+y2: Web introduction complex numbers are imaginary numbers, and the complex plane represents these numbers. We can join this point to the origin with a line segment. Web ⇒ the argument of a complex number is the angle its corresponding vector makes with the positive real axis. | z | = a 2.
Example 13 Find modulus, argument of (1 + i)/(1 i) Examples
⇒ also see our notes on: Web ⇒ the argument of a complex number is the angle its corresponding vector makes with the positive real axis. Web modulus and argument definition any complex number z z can be represented by a point on an argand diagram. Themodulusofzis 6 z=x+ iyy u 3 jzj =r=px2+y2: Find the modulus and argument of.
SM4C Modulus Argument Form of a Complex Number YouTube
Theargumentofzis x re y argz= = arctan:. Modulus ( magnitude ) the modulus or magnitude of a complex number ( denoted by ∣z∣ ), is the distance between the origin and that number. | z | = a 2 + b 2 | 3 + 3 3 i | = 3 2 + ( 3 3) 2 | 3 +.
PPT Complex Numbers PowerPoint Presentation, free download ID956714
Web the modulus and argument are fairly simple to calculate using trigonometry. Web the modulus (also known as the magnitude or absolute value) of a complex number is a scalar value that represents the distance of the complex number from the origin on the. There are, however, other ways to write a complex number, such as in modulus. Web ⇒.
Themodulusofzis 6 Z=X+ Iyy U 3 Jzj =R=Px2+Y2:
Modulus ( magnitude ) the modulus or magnitude of a complex number ( denoted by ∣z∣ ), is the distance between the origin and that number. Web the modulus is the length of the line segment connecting the point in the graph to the origin. The complex number is said to be in cartesian form. There are, however, other ways to write a complex number, such as in modulus.
| Z | = A 2 + B 2 | 3 + 3 3 I | = 3 2 + ( 3 3) 2 | 3 + 3 3 I |.
Using the formula, we have: We can join this point to the origin with a line segment. (b) hence simplify each of the. Web when an argument is outside , add or subtract multiples of until the angle falls within the required range.
Web Introduction Complex Numbers Are Imaginary Numbers, And The Complex Plane Represents These Numbers.
Among the two forms of these numbers, one form is z = a + bi, where i. I) 1 + i tan θ, ii) 1 + i cot θ, iii) 1 sin θ + 1 cos θ i. Web ⇒ the argument of a complex number is the angle its corresponding vector makes with the positive real axis. Web the modulus and argument are fairly simple to calculate using trigonometry.
Theargumentofzis X Re Y Argz= = Arctan:.
The formula |z| = √ (x 2 +y 2 ) gives the modulus of a complex number z = x + iy, denoted by |z|, where x is the real component and y is the. The complex number is said to be in cartesian form. Examples of finding the modulus and argument The complex number z = 4 + 3i.