Parametric Equations In Rectangular Form
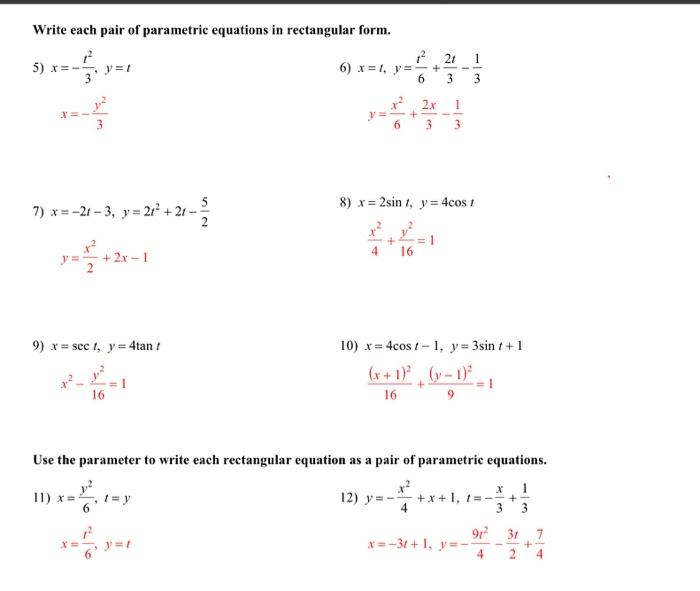
Parametric Equations In Rectangular Form - Web find parametric equations for curves defined by rectangular equations. Y = 3x3 + 5x +6. State the domain of the rectangular form. By convention, t is frequently used as the parameter, though other variables can be used as well. X = t2 x = t 2. Web convert x^2 + y^2 = 1 to parametric form. Web how do you convert each parametric equation to rectangular form: Given \(y=f(x)\), the parametric equations \(x=t\), \(y=f(t)\) produce the same graph. Consider the path a moon follows as it orbits a planet, which simultaneously rotates around the sun, as seen in (figure). You have to solve for \(t\) in one of the equations.
Web in the rectangular coordinate system, the rectangular equation y = f ( x) works well for some shapes like a parabola with a vertical axis of symmetry, but in precalculus and the review of conic sections in section 10.0, we encountered several shapes that could not be sketched in this manner. Web converting parametric equation to a cartesian equation or rectangular form involves solving for t in terms of x and then plugging this into the y equation. Web finding parametric equations for curves defined by rectangular equations. To convert this rectangular equation to parametric form, we make use of our knowledge of trigonometry and its identities. We’re given a pair of parametric equations, and we’re asked to convert this into the rectangular form. Web write down the following mentioned rectangular equations into parametric form. Write the parametric equations in rectangular form and identify the interval for x or y line example show more. X = t2 x = t 2. By convention, t is frequently used as the parameter, though other variables can be used as well. Analysis as values for t progress in a positive direction from 0 to 5, the.
By convention, t is frequently used as the parameter, though other variables can be used as well. Y = x^2+6x + 9 + 5. When we parameterize a curve, we are translating a single equation in two variables, such as x and y ,into an equivalent pair of equations in three variables, x, y, and t. Y = 3x 3 + 5x +6; Web this is an equation for a parabola in which, in rectangular terms, x is dependent on y. Web find parametric equations for curves defined by rectangular equations. Eliminate the parameter from the parametric equations below, and convert the equation into rectangular form. Parametric equations primarily describe motion and direction. At any moment, the moon is located at a. Let’s evaluate the equation 1:
Precalculus Chapter 6.3 Exercises 1118 Convert Parametric Equations
4.2k views 2 years ago parametric equations. Y = 3x3 + 5x +6. Web converting parametric equations to rectangular: Web converting between rectangular and parametric equations. Web parametric equations are a set of equations that express a set of quantities as explicit functions of a number of independent variables, known as parameters. for example, while the equation of a circle.
Rectangular Form Of Parametric Equations akrisztina27
Given \(y=f(x)\), the parametric equations \(x=t\), \(y=f(t)\) produce the same graph. Web converting parametric equation to rectangular form. To convert parametric equations to rectangular form, we need to find a way to eliminate the 𝑡. Y = x 4 + 5x 2 +8; Converting from rectangular to parametric can be very simple:
Rectangular Form Of Parametric Equations akrisztina27
At any moment, the moon is located at a. We’re given a pair of parametric equations, and we’re asked to convert this into the rectangular form. Y = x^2+6x + 9 + 5. When we parameterize a curve, we are translating a single equation in two variables, such as x and y ,into an equivalent pair of equations in three.
Rectangular Form Of Parametric Equations akrisztina27
Web in the rectangular coordinate system, the rectangular equation y = f ( x) works well for some shapes like a parabola with a vertical axis of symmetry, but in precalculus and the review of conic sections in section 10.0, we encountered several shapes that could not be sketched in this manner. Web how do you convert each parametric equation.
SOLVEDFind a rectangular equation equivalent to the given pair of
Parametric equations primarily describe motion and direction. Y = (x+3)^2 + 5. Web converting between rectangular and parametric equations. Following steps must be followed in order to convert the equation in parametric form. From the curve’s vertex at (1, 2), the graph sweeps out to the right.
How to convert parametric equations to rectangular form example 3 YouTube
In this section, we consider sets of equations given by the functions x(t) and y(t), where t is the independent variable of time. Web convert x^2 + y^2 = 1 to parametric form. Remember, this means we need to rewrite this as an equation in terms of 𝑥 and 𝑦. By convention, t is frequently used as the parameter, though.
Parametric Equations Rectangular Form YouTube
Web how do you convert each parametric equation to rectangular form: Y = 3x 3 + 5x +6; Rewrite the equation as t2 = x t 2 = x. At any moment, the moon is located at a. Web writing parametric equations in rectangular form
Rectangular Form Of Parametric Equations akrisztina27
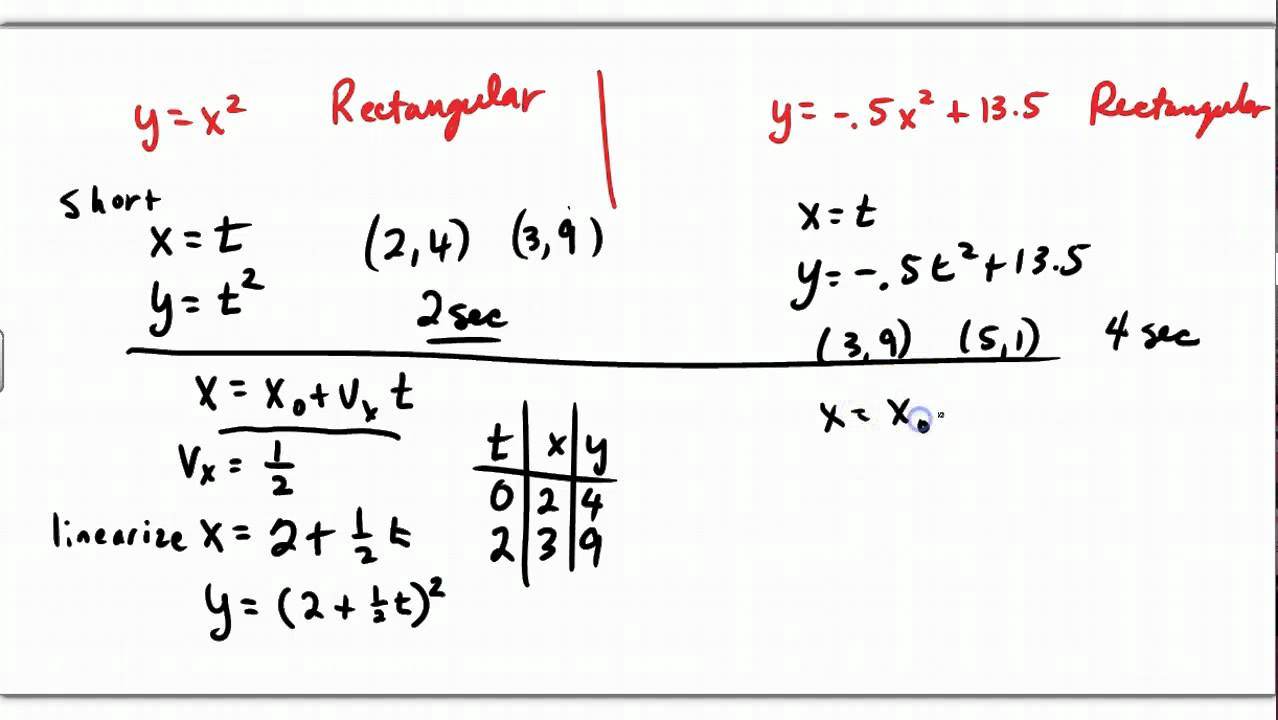
T2 = x t 2 = x. Parametric equations primarily describe motion and direction. Remember, this means we need to rewrite this as an equation in terms of 𝑥 and 𝑦. Web example 1 sketching the graph of a pair of parametric equations by plotting points sketch the graph of the parametric equations x(t) = t2 + 1, y(t) =.
Rectangular Form Of Parametric Equations akrisztina27
Web for the following exercises, convert the parametric equations of a curve into rectangular form. Web converting parametric equation to rectangular form. Write the parametric equations in rectangular form and identify the interval for x or y line example show more. We’re given a pair of parametric equations, and we’re asked to convert this into the rectangular form. Eliminate the.
Rectangular Form Of Parametric Equations akrisztina27
Y = (x+3)^2 + 5. Web convert the parametric equations 𝑥 is equal to the cos of 𝑡 and 𝑦 is equal to the sin of 𝑡 to rectangular form. State the domain of the rectangular form. Web in the rectangular coordinate system, the rectangular equation y = f ( x) works well for some shapes like a parabola with.
X = T2 X = T 2.
Given \(y=f(x)\), the parametric equations \(x=t\), \(y=f(t)\) produce the same graph. Although we have just shown that there is only one way to interpret a set of parametric equations as a rectangular equation, there are multiple ways to interpret a rectangular equation as a set of parametric equations. Y = 3x3 + 5x +6. For parametric equations, put x = t so, the equation becomes, y = 3t 3 + 5t + 6
Web Finding Parametric Equations For Curves Defined By Rectangular Equations.
Web converting parametric equation to rectangular form. Rewrite the equation as t2 = x t 2 = x. Web parametric equations are a set of equations that express a set of quantities as explicit functions of a number of independent variables, known as parameters. for example, while the equation of a circle in cartesian coordinates can be given by r^2=x^2+y^2, one set of parametric equations for the circle are given by x = rcost (1) y. Write the parametric equations in rectangular form and identify the interval for x or y line example show more.
Let’s Evaluate The Equation 1:
We have 𝑥 is equal to some function of 𝑡 and 𝑦 is equal to some other function of 𝑡. X = t2 x = t 2 , y = t9 y = t 9. Y = (x+3)^2 + 5. We’re given a pair of parametric equations, and we’re asked to convert this into the rectangular form.
You Have To Solve For \(T\) In One Of The Equations.
Web a typical parametric equation will be in the form x = f ( t) and y = g ( t). Web for the following exercises, convert the parametric equations of a curve into rectangular form. This video explains how to write a parametric equation as an equation in rectangular form. Web writing parametric equations in rectangular form